
Every time Zong Chuanming begins to isolate himself from all social activities, people familiar with him can roughly guess that he is working on something big.
Mathematical research is known for its abstraction and often difficult for laymen to understand. But even some problems that seem natural and simple can also baffle the world’s smartest minds. For example, what shapes of tiles can cover the whole plane without gaps, is such a problem.
Recently, a research result by Prof. Zong Chuanming from Tianjin University’s School of Mathematics was published in the fifth issue of the Notices of the American Mathematical Society in 2020 as the recommended paper, in which a series of complete answers to the classical tiling problems in mathematics were given.
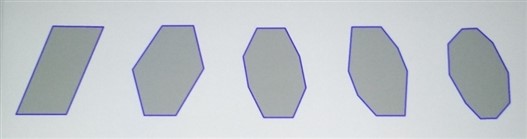
Five types of convex polygons that can form fivefold translative tilings in the plane
Century Puzzle
As early as in ancient Greece, philosophers such as Aristotle and Archimedes discovered some paving compositions; in 1619, Johannes Kepler made a complete classification of Archimedean tilings; in 1885, Russian scientist Fedorov systematically studied the most regular tiling and laid the foundation for the crystallography theory.
However, the progress seemed to slow down when German mathematician Bieberbach first proposed the classification of two-dimensional identical convex pentagons around 1916.
“Such a seemingly simple geometric problem has baffled many outstanding mathematicians.” Prof. Zong said, “The research process of Bieberbach’s problem is extremely complicated and tortuous. It has been wrongly declared to be completely solved at least three times in the past century.”
Apart from the systematic research of mathematicians and computer experts, some amateurs have made amazing contributions to the process of looking for the two-dimensional identical convex domains. For example, Marjorie Rice, who took only one year of math in high school, found four types of new pentagon tiles in 1978. For this reason, a lobby floor of the Mathematical Association of America in Washington is paved with one of Rice’s pentagon tiles in her honor.
After a century, in addition to triangles and quadrilaterals, three kinds of hexagon and fifteen types of pentagons polygon tiles have been found. The last kind of pentagon tiling was discovered by three European and American mathematicians using computers in 2016.
After Six Years without Progress
Mathematicians always like to ask themselves difficult questions. Since the 1930s, many outstanding mathematicians, such as P. Furtwängler, G.Hajo´s, R. M. Robinson, begun to study multiple tilings, and have made a series of important achievements. By natural superposition, single tilings can produce any number of multiple layer tilings. But what mathematicians want to know is, apart from the quadrilaterals and hexagons discovered by Fedorov, what other geometric shapes can form two-, three-, or multiple translative tilings?
This aroused Prof. Zong’s interest. In 2006, he published a monograph in Cambridge University Press, which introduced the achievements of the above three people, and began to organize graduate students to study and discuss their related work once a week, trying to make a breakthrough in classification.
However, six years’ work brought them nowhere on this issue.
In 2012, three European and American scholars found an octagon that can form seven-fold translative tilings, reviving the research enthusiasm of Prof. Zong’s team. “We redesigned the research plan. On the one hand, my graduate students use computers to find better examples of multiple tilings; on the other hand, I use the achievements and thinking methods accumulated in the accumulation theory for many years to focus on the study of low multiple lattice tilings.”
After years of hard work, amid many failures, Zong Chuanming and his students finally ushered in unexpected gains around 2017.
First of all, they proved that, besides the two kinds of polygons discovered by Fedorov, there is no other convex domain that can form any two-, three-, or fourfold translative tiling; then, they found one type of decagon that can form fivefold translative tiling; and by gradually implementing the pre-designed research scheme, Prof. Zong independently proved that there are only five convex polygons that can form fivefold translative tilings: parallelograms, centrally symmetric hexagons, two types of special octagons and one type of very special decagon.
“We published these results on arXiv. In the next few days, I received many congratulations from my European and American counterparts.” Prof. Zong said frankly that the intense interest showed by the foreign counterparts not only brought them the joy of success, but also the huge pressure of competition.
He decided to work harder and finish the story.
Treat mathematical research like works of art
“Multiple lattice tiling is the most important and special type of multiple translative tilings, but a perfect theory should include all multiple translative tilings,” Prof. Zong Chuanming realized that innovative thinking was key to solve such a long-standing problem.
After careful consideration, he designed a systematic research scheme, introducing a combined counting formula at each tiling vertex. Next, he organized the research team to implement the program in blocks.
During this period, Prof. Zong declined all meetings, reviews, reports and other activities to focus on his research plan.
In 2018, Prof. Zong and his doctoral student Qi Yang finally realized his proof plan. They found and proved that, except for the two kinds of polygons discovered by Fedorov, there is no other convex domain that can form any two-, three-, or fourfold translative tiling; the fivefold translative tiling must be fivefold lattice tiling, and only parallelograms, centrally symmetric hexagons, two types of special octagons and one type of very special decagon can realized it.
Later, Zong Chuanming completely depicted all convex polygons that can form sixfold lattice tiling: parallelograms, central symmetrical hexagons, one type of special octagon and two types of very special decagons.
His research results were published in the Notices of the American Mathematical Society in May 2020, and became the magazine’s first “recommended paper” written by a Chinese scholar in the past five years.
The paper was highly spoken of by multiple famous scholars, such as Ziegler, President of Berlin University, Morgan, former vice president of American Mathematical Association and so on. Not long ago, Tian Gang, an academician of the Chinese Academy of Sciences and President of the Chinese Mathematics Association, mentioned this work in his lecture inside and outside mathematics.
“Mathematical studies is like artistic creation sometimes, and we always hope to complete some eternal works like the masterpieces of Leonardo da Vinci or Michelangelo. Though our work is far from a towering achievement in the world of mathematics, it is a complete and fundamental achievement though, like a small piece of tiling stone in the great hall of mathematics.”
Just as Zong Chuan-ming said, the achievements of mathematical research can be eternal. Behind the splendid mathematical achievements, the stories of the determination, striving, failure, obsessiveness, and enlightenment of mathematicians will be passed down to and appreciated by future generations.




